Maths
Subject Leaders - Miss Robinson, Miss Alexander & Mr Leeman

At RAB, we inspire children to appreciate the beauty and power of mathematics and we have the determination that all children will become successful mathematicians during their time in the school. We recognise that mathematics is a highly creative, inter-connected discipline, essential in laying down the foundations for understanding the world and skills for life.
By the end of the mathematical journey a child embarks on at RAB, they will have become more flexible and fluent thinkers; inquisitive and insightful questioners; attentive and deliberate observers; and resourceful and resilient problem solvers.
Within our challenging and engaging lessons, conceptual understanding is prioritised over procedural understanding, allowing children to think deeply and articulate their reasoning.
From an early age, children gain a sense of number and are taught key fluency facts that underpin the rest of the curriculum. By having instant recall of these facts, children can wrestle with increasingly complex problems that form rich connections between areas of the curriculum. Please see details of Number Fluency & Times Tables: The RAB Way and Fluency in Multiplication & Division at RAB: A Summary below.
At RAB, we follow a mastery curriculum. We believe that when teaching mathematics, it is important that lessons are broken into small steps so that all children can become successful mathematicians. Daily masterclasses are used in the afternoon to ensure that any learner who needs more practise is ready for the next set of small steps the following day.
how we teach maths at rab
Throughout R A Butler’s mathematics curriculum, you should expect to see:
• Whole class together – Our mathematics is taught within mixed ability groups. Occasionally, support and extension groups will work within small groups outside of the classroom with a trained LSA. In year 6, mathematics is taught amongst three smaller mixed ability groups and one independent group. All groups will do the same lessons but the independent group will have less support in the room.
• Longer but deeper – in order to address the aims of the national curriculum, our long/medium term plans have been adjusted to allow longer on topics. Each lesson focus is on one key conceptual idea and connections are made across mathematical topics.
• Key learning points are identified during planning (collaboratively in year groups) and a clear journey through the maths should be shown within planning and on IWB slides (also reflected on learning walls). Greater depth activities will be planned carefully to ensure rapid graspers learning is stretched and challenged without crossing into future year’s curriculum content.
• Questions will probe pupil understanding throughout and responses are expected to be framed within full sentences using precise mathematical vocabulary. Stem sentences may be used to scaffold children’s reasoning, vocabulary and understanding.
• ‘Misconceptions’ are identified during the planning process and children will be supported through these. We celebrate mistakes to ensure children understand that this is the way we learn. Mistakes are celebrated, addressed and overcome together.
• Develop reasoning and deepen understanding (contexts and representations of mathematics) – problems are usually set in real-life contexts - carefully chosen representations (manipulatives and images) are used by all to explore concepts. Reasoning statements may also be displayed in the classroom to support children answer and writing in full mathematical sentences.
• Step by step approach – journey through the mathematics in small easy to digest steps. The smart notebook/PowerPoints/planning clearly show this step-by-step approach. A wide range of materials (including the NCETM spines) are used to supplement the material produced by the White Rose.
• Discussion and feedback – pupils have opportunities to talk to their partners and explain/clarify their thinking throughout the lesson but may expected to complete some written work independently.
• Reflecting – children will reflect on their successes within the lesson using the feedback 3 and 5 systems
• Practising - not drill and practice but practice characterised by variation
• Marking and Feedback – we have a whole school marking policy which is followed within every classroom. Marking should reflect feedback and pupils will have opportunities to respond to this. Same day masterclasses are used to ensure all children are ready for the next small step.
• SEN pupils – may be supported by additional adults, different resources, differentiated activities. They may also complete additional activities outside of the mathematics lesson. Additionally, challenging activities may also be planned for children who are exceeding the curriculum expectations.
• Talk in maths - by orally clarifying their thinking, pupils consolidate their understanding. Maths talk is supported through a shared framework of sentence prompts and high expectations and modelled explanations. Listening to pupils’ discussion and explanations provides an invaluable assessment opportunity. STEM sentences will be carefully planned and used within lessons to encourage children’s oracy, answering in full sentences.
• Use of resources, models and images - all pupils benefit from the appropriate use of resources to support their understanding of mathematical concepts. Teachers ensure that pupils can move from tangible experiences before moving to the abstract. This applies to pupils of all ages and attainment.
• Challenge for all - all pupils should experience maths learning which challenges and stretches them. Pupils should be encouraged to develop their resilience and teachers should plan activities where outcomes are not limited. All pupils in a class should experience a similar amount of success.
• Depth before breadth - the National Curriculum is a mastery curriculum whereby the expectation is that the majority of a class will have learnt and understood the objectives by the end of the year. Extension is through enrichment, reasoning and depth of understanding. There is not an expectation to move to the subsequent year’s teaching programme.
• Assessment for learning - this is an integral part of all lessons. Teachers are expected to respond to pupils within a lesson and adjust planning and teaching to meet pupils’ needs.
Mastery at rab
What is Teaching for Mastery at RAB?
At R A Butler, we follow a mastery curriculum (White Rose) and every lesson will demonstrate the 5 key strands to mastery as defined by the NCETM.
The five key strands of mastery are:
- Coherence
- Mathematical Thinking
- Fluency
- Structures and Representations
- Variation
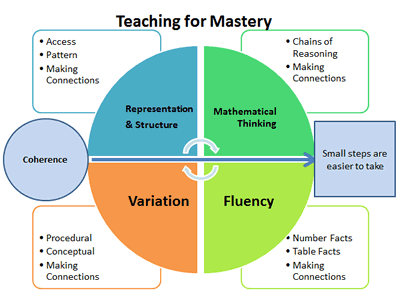
Coherence
Coherence is the idea that the maths curriculum is carefully sequenced in a manner such that the learning builds upon previous knowledge and broken into small easy to manage steps. Therefore the learning becomes easier for the children to grasp and everyone is able to achieve within a lesson. This then will lead to all children being able to move through the mathematics curriculum at roughly the same pace.
Mathematical Thinking
Mathematical thinking is ensuring that we are giving children the chance to think like mathematicians in all of our lessons. We will promote the children in verbalising their understanding of concepts and provide them with the chance to fully explain problems that they face. We will also promote our mathematicians to spot patterns and explain what they are noticing happen. This will allow them to become more efficient problem solvers.
Fluency
Having secure knowledge of the key instant recall facts is an essential part of becoming an efficient mathematician. It means that the child can focus their entire attention on the mathematics behind a problem rather than try to calculate facts that should be instantly recalled.
When these facts are fluent, we can then teach children how to become more efficient through the use of multiple methods and help them select the most appropriate mental method for any situation. This is key in helping our mathematicians becoming as flexible and agile as possible.
Structures and Representations
The key to our children becoming successful mathematicians is that they develop a strong and secure conceptual understanding of the mathematics rather than a procedural understanding. One way to ensure this happens is through the use of representations. Children will be exposed to multiple images throughout their time at RAB that help them understand the mathematics behind certain concepts. Before being taught formal written methods, children will have the chance to explore concepts through the use of physical manipulatives and pictorial representations. Once these are understood, children will be able to fully understand the principles behind the abstract methods we teach.
Variation
It is key that we expose the children to concepts in as many ways as possible through the use of different representations and structures. This will allow them to become more flexible during the problem solving process and have a wide range of strategies that they can deploy.
Variation is also about being really explicit about what we want the children to attend to. In our lessons, we will expose the children to not only what the key features of a concept are but also what that concept is not. Through the use of standard, non-standard and non-examples, we can provide our children with a really deep understanding of the mathematics that we are teaching them.
fluency at rab
In order to be a successful mathematician, we recognise the importance of being fluent with key number facts. These include number bonds, addition and subtraction facts within 20 and times tables up to 12 x 12. Throughout mathematics at R A Butler, children are continually exposed to the teaching of these facts rather than the testing of facts. Here are some of the ways that we will help your children become fluent with number facts.
Mastering Number
Throughout EYFS and KS1, children have a 10 minute daily lesson called mastering number. This is a scheme produced by the NCETM aiming to ensure that all children are fluent with number bonds and addition/subtraction facts before the end of KS1. Within these sessions, children are exposed to structures of number and taught how to manipulate these to become more flexible. Often these sessions will be taught using a Rekenrek (see the picture below).

Numbots
Numbots is a program designed to help KS1 children practice their fluency facts that they are learning in class. All children in years 1 and 2, will have a log in that they can use and play the games to help them develop their number sense.
Times Tables
It is vital that children are fluent with multiplication facts in order to help them solve mathematical problems accurately and confidently. The national curriculum specifies that children should be able to recall times tables facts up to 12 x 12 by the end of year 4. In the summer of year 4, schools are required to administer an online multiplication check to see whether this has been met. Consolidation for all children will happen during years 5 and 6 as these number facts underpin a huge amount of the year 5 and 6 curriculum.
By the end of year 2, we would expect all children to be fluent (answer within 5 seconds) with their 2s, 5s and 10s.
By the end of year 3, we would expect all children to be fluent in 3s, 4s, 8s and 6s.
By the end of year 4, we would expect all children to be fluent in 9s, 11s, 7s and 12s.
Children are exposed to structures of multiplication and taught multiple representations of each table. In order to practice their tables, children are expected to use our online platform, Times Table Rockstars (TTRS).
This program has been developed over many years and has in-built algorithms which ensure that children are practising the exact number fact that they need to be practising in order to become fluent.
In years 2 – 6, we run a times table reward scheme, where children are challenged to become ultimate rockstars in their journey of learning their tables. Regularly, Andre Ballard (a world renowned rockstar) will visit assembly to get updates in how the children are doing in their tables! He may even sign a few certificates!
